Tests of Linear Combinations of Means, Independent
Groups (3 of 7)
-
The fourth step is to calculate the
probability of a value of L this different or more different from the
value specified in the null hypothesis (zero). Applying the
general formula, one obtains
where L is the value of the linear combination in the sample and sL is the standard error of L. The formula for the standard error of L is given below.
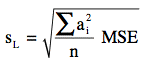
This formula is the same as the formula for σL except that MSE (an estimate of
) is used in place of
. The formula for MSE (and SL) is the same as the formula
used in the calculation of a confidence interval
on a linear combination of means.
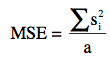
where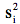
is the sample variance of the ith group and "a" is the number of
groups. This formula assumes homogeneity of
variance and that the "a" sample
sizes are equal. A related formula is described
elsewhere that can be used with unequal
sample sizes.
The degrees of freedom are equal to the sum of the degrees of freedom in the a = 3 groups. Therefore, df = a(n-1) = 3(4-1) = 9. A t table shows that the two-tailed probability value for a t of 2.33 with 9 df is 0.045.