Tests of Differences between Proportions (2 of 5)

---

2. The second step is to choose a significance level. Assume the 0.05 level is chosen.
   
   
3. The third step is to compute the difference between the sample proportions. In this example, p₁ - p₂ = 73/85 - 43/82 = 0.8588 - 0.5244 = 0.3344.
   
   
4. The fourth step is to compute p, the probability (or probability value). It is the probability of obtaining a difference between the proportions as large or larger than the difference observed in the experiment. Applying the general formula to the problem of differences between proportions
   
   
   
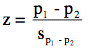
   where p₁- p₂ is the difference between sample proportions and
   
   
   
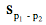
   is the estimated standard error of the difference between proportions. The formula for the estimated standard error is:
   
   
   
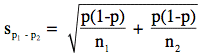
   where p is a weighted average of the p₁ and p₂, n₁ is the number of subjects sampled from the first population, and n₂ is the number of subjects sampled from the second population.