Tests of Pearson's Correlation (5 of 6)
Suppose a theory of intelligence predicts that the correlation between intelligence and spatial ability is 0.50. A sample of 73 high school students was given both a test of intelligence and spatial ability. The correlation between the tests was 0.677. Is the value of 0.677 significantly higher than 0.50? The null hypothesis therefore is ρ = 0.5.
The r to z' table can be used to convert both the r of 0.677 and the of 0.50 to Fisher's z'. The values of z' are 0.824 and 0.549 respectively. The standard error of z' is:
and therefore,
z = (0.824 - 0.549)/0.1195 = 2.30.
A z table shows that the two-tailed probability value for a z of 2.30 is 0.021. Therefore, the null hypothesis that the population correlation is 0.50 can be rejected.
Summary of Computations
- Specify the null hypothesis and an alternative hypothesis.
- Compute Pearson's r
(click here for formula)
- If the null hypothesis is ρ = 0 then compute
t using the following formula:
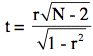
- Use a t table to compute p for t and N-2 df.