Confidence Interval for μ, Standard Deviation Known (2 of 3)
The 10 scores are: 320, 380, 400, 420, 500, 520, 600, 660, 720, and 780. Therefore, M = 530, N = 10, and
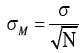 =
= The value of z for the 95% confidence interval is the number of standard deviations one must go from the mean (in both directions) to contain 0.95 of the scores.
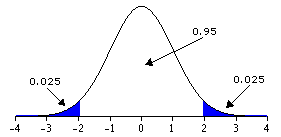
It turns out that one must go 1.96 standard deviations from the mean in both directions to contain 0.95 of the scores. The value of 1.96 was found using a z table. Since each tail is to contain 0.025 of the scores, you find the value of z for which 1-0.025 = 0.975 of the scores are below. This value is 1.96.
All the components of the confidence interval are now known:
M = 530, σM = 31.62, z = 1.96.
Lower limit = 530 - (1.96)(31.62) = 468.02
Upper limit = 530 + (1.96)(31.62) = 591.98