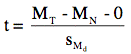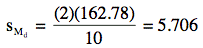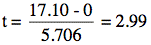Tests of Differences between Means, Independent Groups, Standard Deviation
Estimated (4 of 8)


- (continued) For the present example,
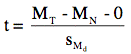
MSE = (81.54 + 244.03)/2 = 162.78,
and
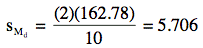
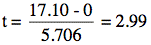
The probability value for t can be determined using a
t table. The degrees of freedom for t is
equal to the degrees of freedom for MSE which is equal to df =
n1 - 1 + n2 -1 = 18 or, df = N - 2 where N =
n1 + n2 The probability is: p = 0.008.
- In step 5, the probability computed in Step
4 is compared to the significance level stated in Step 2. Since the
probability value (0.008) is less than the significance level (0.05)
the effect is significant.
- Since the effect is significant,
the null hypothesis is rejected. It is concluded that the mean memory
score for experts is higher than the mean memory score for novices.
- A report of this experimental result
might be as follows:
The mean number of pieces recalled by tournament
players (MT = 63.89) was significantly higher than the
mean number of pieces recalled by novices (MN = 46.79),
t(18) = 2.99, p = 0.008.
The expression "t(18) = 2.99" means that a t test with 18 degrees
of freedom was equal to 2.99. The probability value is given by "p =
0.008." Since it was not mentioned whether the test was one- or two-tailed,
it is assumed the test was two tailed.