Confidence Interval on Pearson's Correlation (2 of 3)

---

From the general formula for a confidence interval, the formula for a confidence interval on z' is:



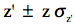
which equals



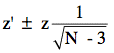
A z table can be used to find that for the 99% confidence interval you should use a z of 2.58. For N = 100, the standard error of z' is 0.102.

The confidence interval for z' can be computed as:

Lower limit = 0.69 -(2.58)(0.102) = 0.43
Upper limit = 0.69 +(2.58)(.102) = 0.95

Using the r to z' table to convert the values of 0.43 and 0.95 back to Pearson r's, it turns out that the confidence interval for the population value of Pearson's correlation, rho (ρ) is:

0.41 ≤ ρ ≤ 0.74

Therefore, the correlation between SAT scores and college grades is highly likely to be somewhere between 0.41 and 0.74.