Confidence Interval for a Proportion (2 of 3)
The value of p is 12/40 = 0.30. The estimated value of σp is
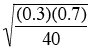= 0.072. A z table can be used to determine that the z for a 95% confidence interval is 1.96. The limits of the confidence interval are therefore:
Lower limit = .30 - (1.96)(0.072) = .16Correction for Continuity
Upper limit = .30 + (1.96)(0.072) = .44.
The confidence interval is: 0.16 ≤ π ≤ .44.
Since the sampling distribution of a proportion is not a continuous distribution, a slightly more accurate answer can be arrived at by applying the correction for continuity. This is done simply by subtracting 0.5/N from the lower limit and adding 0.5/N to the upper limit. For the present example, 0.5/N = 0.5/40 = 0.01. Therefore the corrected interval is: 0.15 ≤ π ≤ 0.45.